Home » Course Layouts » Free Course Layout Udemy
This graduate-level course focuses on one-dimensional nonparametric statistics developed mainly from around 1945 and deals with order statistics and ranks, allowing very general distributions.
0
2
English
English [CC]
- Learn basic syntax that can apply to any language.
- Learn what is a programming language and the basic concepts for beginners.
- Understand what is Javascript in it's truest form.
- Know the basic syntax of Javascript.
- Know some hidden quirks in Javascript.
Description
For multidimensional nonparametric statistics, an early approach was to choose a fixed coordinate system and work with order statistics and ranks in each coordinate. A more modern method, to be followed in this course, is to look for rotationally or affine invariant procedures. These can be based on empirical processes as in computer learning theory.
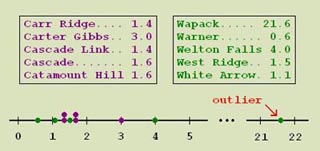
Robustness, which developed mainly from around 1964, provides methods that are resistant to errors or outliers in the data, which can be arbitrarily large. Nonparametric methods tend to be robust.
Course content
- Outliers Unlimited
- Quantiles Unlimited
- Combining the Run and Mann-Whitney-Wilcoxon Tests Unlimited
- Bretagnolle and Massart’s Proof of the KMT Theorem for the Uniform Empirical Process Unlimited
- The Delta-Method and Asymptotics of some Estimators Unlimited
- Introduction to Robustness: Breakdown Points Unlimited
- Breakdown Points of some 1-Dimensional Location Estimators Unlimited
- M-estimators and their Consistency Unlimited
- The Spatial Median Unlimited
- Non-existence of some Affinely Equivariant Location Functionals in Dimension d ≥ 2 Unlimited
- Location and Scatter Functionals Unlimited
Instructor
