Home » Course Layouts » Free Course Layout Udemy
This is a undergraduate course
0
English
English [CC]
FREE
- Learn basic syntax that can apply to any language.
- Learn what is a programming language and the basic concepts for beginners.
- Understand what is Javascript in it's truest form.
- Know the basic syntax of Javascript.
- Know some hidden quirks in Javascript.
Description
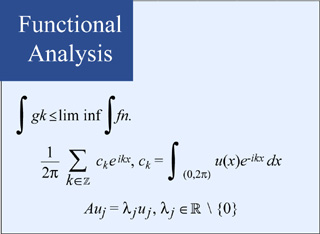
It will cover normed spaces, completeness, functionals, Hahn-Banach theorem, duality, operators; Lebesgue measure, measurable functions, integrability, completeness of L-p spaces; Hilbert space; compact, Hilbert-Schmidt and trace class operators; as well as spectral theorem.
Course content
- Linear spaces, metric spaces, normed spaces Unlimited
- Linear maps between normed spaces Unlimited
- Banach spaces Unlimited
- Lebesgue integrability Unlimited
- Lebesgue integrable functions form a linear space Unlimited
- Null functions Unlimited
- Monotonicity, Fatou’s Lemma and Lebesgue dominated convergence Unlimited
- Hilbert spaces Unlimited
- Baire’s theorem and an application Unlimited
- Bessel’s inequality Unlimited
- Closed convex sets and minimizing length Unlimited
- Compact sets. Weak convergence. Weak compactness Unlimited
- Baire’s theorem. Uniform boundedness. Boundedness of weakly convergent sequences Unlimited
- Fourier series and L2 Unlimited
- Open mapping and closed graph theorems Unlimited
- Bounded operators. Unitary operators. Finite rank operators Unlimited
- The second test Unlimited
- Compact operators Unlimited
- Fredholm operators Unlimited
- Completeness of the eigenfunctions Unlimited
- Dirichlet problem for a real potential on an interval Unlimited
- Dirichlet problem (cont.) Unlimited
- Harmonic oscillator Unlimited
- Completeness of Hermite basis Unlimited
- The fourier transform on the line Unlimited
- Hahn-Banach and review Unlimited
Instructor
Massachusetts Institute of Technology
5
5
1
1916
1520
